CIE International A-Level Maths: Pure Mathematics 2/3

Focused View

Woody Lewenstein

21:58:17

58 View

1. Introduction

1. Introduction.mp4

01:43

2. Whats the Difference Between Paper 2 and Paper 3.mp4

01:10

2. Algebra

1. The Modulus Function - Graphs - Part 1.mp4

10:02

2. The Modulus Function - Graphs - Part 1 - quiz.html

3. The Modulus Function - Graphs - Part 2.mp4

08:30

4. The Modulus Function - Graphs - Part 2 - quiz.html

5. The Modulus Function - Equations and Inequalities - Part 1.mp4

11:26

6. The Modulus Function - Equations and Inequalities - Part 1.html

7. The Modulus Function - Equations and Inequalities - Part 2.mp4

07:47

8. The Modulus Function - Equations and Inequalities - Part 2.html

9. Polynomial Division.mp4

13:41

10. Polynomial Division Intro - quiz.html

11. Polynomial Division - Examples - 1.mp4

12:11

12. Division Examples - part 1 - quiz.html

13. Polynomial Division - Examples - 2.mp4

12:22

14. Division Examples - part 2 - quiz.html

15. Remainders - Part 1.mp4

06:28

16. Remainders - part 1 - quiz.html

17. Remainders - Part 2.mp4

10:04

18. Remainders - part 2 - quiz.html

19. The Factor Theorem.mp4

17:23

20. The Factor Theorem - quiz.html

21. The Long Division Method.mp4

13:58

22. The Long Division Method - quiz.html

3. Logarithms and Exponential Functions

1. Exponential Functions - Intro.mp4

10:27

2. Exponentials Introduction - quiz.html

3. THE Exponential Function.mp4

12:08

4. THE Exponential Function - quiz.html

5. Differentiating e^x.mp4

07:56

6. Differentiating e^x - quiz.html

7. Exponential Modelling.mp4

15:27

8. Exponential Modelling - quiz.html

9. Logarithms - Intro.mp4

09:32

10. Logarithms Introduction - quiz.html

11. Simplifying Logarithms.mp4

06:43

12. Logarithms Laws.mp4

07:40

13. Using Log Laws.mp4

08:11

14. Using the Log Laws - quiz.html

15. Logarithmic Equations - Part 1.mp4

10:35

16. Solving Logarithmic Equations - part 1 - quiz.html

17. Logarithmic Equations - Part 2.mp4

13:47

18. Solving Logarithmic Equations - part 2 - quiz.html

19. The Natural Logarithm.mp4

09:20

20. The Natural Log - quiz.html

21. Logarithmic Graph.mp4

05:22

22. Graphs of Logarithms - quiz.html

23. Non-Linear Data - Exponential Models.mp4

09:48

24. Non-Linear Data - Exponential Models - quiz.html

25. Non-Linear Data - Polynomial Models.mp4

14:57

26. Non-Linear Data - Polynomial Models - quiz.html

27. Non-Linear Data - Exam Questions.mp4

10:57

28. Non-Linear Data - Exam Question - quiz.html

4. Trigonometry

1. Sec, Cosec and Cot.mp4

13:00

2. Graphs of Sec, Cosec and Cot.mp4

15:03

3. Graphs of Sec, Cosec and Cot - quiz.html

4. Sec, Cosec and Cot - Simple Identities.mp4

09:56

5. Sec, Cosec and Cot - Simple Equations.mp4

12:11

6. Sec, Cosec and Cot - Simple Equations - quiz.html

7. The Pythagorean Identities.mp4

04:36

8. The Pythagorean Identities - quiz.html

9. The Pythagorean Identities - Proving Identities.mp4

10:31

10. The Pythagorean Identities - Proving Identities - quiz.html

11. The Pythagorean Identities - Solving Equations.mp4

11:59

12. The Pythagorean Identities - Solving Equations - quiz.html

13. The Pythagorean Identities - Exam-Style Problems.mp4

12:13

14. The Pythagorean Identities - Exam Style Problems - quiz.html

15. Inverse Trig Functions - Graphs.mp4

12:47

16. Inverse Trig Functions - Graphs - Quiz.html

17. Inverse Trig Functions - Calculations.mp4

07:06

18. Inverse Trig Functions - Calculations - quiz.html

19. The Addition Formulae.mp4

14:13

20. Using The Addition Formulae.mp4

11:08

21. Using the Addition Formulae - quiz.html

22. The Double Angle Formulae.mp4

10:54

23. The Double Angle Formulae - Solving Equations - Part 1.mp4

04:39

24. The Double Angle Formulae - Solving Equations - Part 1.html

25. The Double Angle Formulae - Solving Equations - Part 2.mp4

07:07

26. The Double Angle Formulae - Solving Equations - Part 2.html

27. The Double Angle Formulae - Proving Identities.mp4

10:07

28. The Double Angle Formulae - Proving Identities - quiz.html

29. The Double Angle Formulae - Exam-Style Problems.mp4

13:26

30. The Double Angle Formulae - Exam-Style Problems - quiz.html

31. Harmonic Form acos(x) + bsin(x).mp4

14:32

32. Harmonic Form acosx + bsinx.html

33. Harmonic Form - Solving Equations.mp4

06:27

34. Harmonic Form - Solving Equations - quiz.html

35. Harmonic Form - Minimum and Maximum Points - Part 1.mp4

07:48

36. Harmonic Form - Minimum and Maximum - Part 1 - quiz.html

37. Harmonic Form - Minimum and Maximum Points - Part 2.mp4

07:42

38. Harmonic Form - Minimum and Maximum - Part 2 - quiz.html

39. Modelling With Trigonometric Equations.mp4

11:42

40. Modelling With Trig Equations - quiz.html

5. Differentiation

1. Differentiating Sin(x) and Cos(x).mp4

15:33

2. Differentiating Sinx and Cosx - quiz.html

3. Using the Derivatives of Sin(x) and Cos(x).mp4

04:30

4. Using the Derivatives of Sinx and Cosx.html

5. Differentiating Exponential Functions.mp4

04:58

6. Differentiating Exponential Functions - quiz.html

7. Differentiating Ln(x).mp4

05:18

8. Differentiating lnx - quiz.html

9. The Chain Rule - f(x)^n.mp4

11:18

10. The Chain Rule - f(x)^n - quiz.html

11. The Chain Rule - Trigonometric Functions.mp4

04:16

12. The Chain Rule - Trigonometric Functions - quiz.html

13. The Chain Rule - Exponential Functions.mp4

04:18

14. The Chain Rule - Exponential Functions - quiz.html

15. The Chain Rule - Logarithmic Functions.mp4

04:53

16. The Chain Rule - Logarithmic Functions - quiz.html

17. The Product Rule.mp4

12:16

18. The Product Rule - quiz.html

19. The Quotient Rule.mp4

11:36

20. The Quotient Rule - quiz.html

21. Derivatives of Tan, Sec, Cosec and Cot.mp4

10:03

22. Derivatives of Tan, Sec, Cosec and Cot - quiz.html

23. Using the Derivatives of Tan, Sec, Cosec and Cot.mp4

09:58

24. Using the Derivatives of Tan, Sec, Cosec and Cot - quiz.html

25. Implicit Differentiation.mp4

14:50

26. Implicit Differentiation - quiz.html

27. Implicit Differentiation - Stationary and Critical Points.mp4

10:19

28. Implicit Differentiation - Stationary and Critical Points - quiz.html

29. Second Derivatives - Convex and Concave Functions.mp4

12:30

30. Second Derivatives - Convex and Concave Functions - quiz.html

31. Parametric Equations - Introduction.mp4

05:17

32. Converting Parametric Equations to Cartesian Form - Substitution.mp4

02:33

33. Converting Parametric Equations to Cartesian Form - Substitution - quiz.html

34. Converting Parametric Equations to Cartesian Form - Pythagorean Identities.mp4

05:49

35. Converting Parametric Equations to Cartesian Form - Pythagorean Identities - qui.html

36. Converting Parametric Equations to Cartesian Form - Double Angles.mp4

05:07

37. Converting Parametric Equations to Cartesian Form - Double Angle Formulae - quiz.html

38. Intersections of Parametric Equations and Lines.mp4

04:27

39. Intersection of a Parametric Equation with a Line.html

40. Differentiating Parametric Curves.mp4

11:14

41. Differentiating Parametric Equations - quiz.html

42. Differentiating Parametric Curves - Stationary Points.mp4

09:38

43. Differentiating Parametric Equations - Stationary and Critical Points - quiz.html

6. Integration

1. Integrating Standard Functions.mp4

09:01

2. Integrating Standard Functions - quiz.html

3. Integrating f(ax + b).mp4

09:43

4. Integrating f(ax+b) - quiz.html

5. Trigonometric Identities For Integration - Pythagorean Identities.mp4

06:37

6. Trig Identities for Integration - Pythagorean Identities - quiz.html

7. Trigonometric Identities For Integration - Double Angle Formulae.mp4

10:13

8. Trig Identities for Integration - Double Angle Formulae - quiz.html

7. Numerical Solutions of Equations

1. Locating Roots.mp4

15:14

2. Iteration - Part 1.mp4

09:56

3. Iteration - Part 2.mp4

11:11

8. Further Algebra

1. Partial Fractions - Two Factors.mp4

12:54

2. Two Factors - quiz.html

3. Partial Fractions - Three Factors.mp4

14:04

4. Three Factors - quiz.html

5. Partial Fractions - Repeated Factors.mp4

14:43

6. Repeated Factors - quiz.html

7. Partial Fractions - Top Heavy Fractions.mp4

10:02

8. Top Heavy Fractions - quiz.html

9. The Binomial Expansion Formula (When n is Not a Positive Integer).mp4

13:16

10. The Binomial Expansion Formula - quiz.html

11. Using the Binomial Expansion Formula.mp4

12:26

12. Using Binomial Expansion - quiz.html

13. Binomial Expansion When the First Term is Not 1.mp4

07:26

14. When The First Term Is Not 1 - quiz.html

15. Approximations.mp4

11:15

16. Approximations - quiz.html

17. Binomial Expansion - Algebraic Fractions.mp4

09:02

18. Binomial Expansion With Algebraic Fractions - quiz.html

19. Binomial Expansion - Partial Fractions.mp4

09:55

20. Binomial Expansion With Partial Fractions - quiz.html

9. Further Integration

1. Reverse Chain Rule f(x)[f(x)]^n - Part 1.mp4

10:17

2. Reverse Chain Rule f(x)[f(x)]^n - Part 2.mp4

04:54

3. Reverse Chain Rule - f(x)[f(x)]^n - quiz.html

4. Reverse Chain Rule - Exponentials.mp4

08:07

5. Reverse Chain Rule - Exponential Functions - quiz.html

6. Reverse Chain Rule - Logarithmic Functions - Part 1.mp4

05:26

7. Reverse Chain Rule - Logarithmic Functions - Part 2.mp4

07:26

8. Reverse Chain Rule - Logarithmic Functions - quiz.html

9. Mixed Reverse Chain Rule Problems.mp4

05:33

10. Using Partial Fractions For Integration - Part 1.mp4

08:58

11. Using Partial Fractions For Integration - Part 2.mp4

08:35

12. Using Partial Fractions for Integration - quiz.html

13. Integration By Parts - Polynomial x Exponential.mp4

08:25

14. Integration by Parts - Polynomial x Exponential - quiz.html

15. Integration By Parts - Polynomial x Trigonometric.mp4

08:52

16. Integration by Parts - Polynomial x Trigonometric - quiz.html

17. Integration By Parts - Polynomial x Logarithmic.mp4

06:26

18. Integration by Parts - Polynomial x Logarithmic - quiz.html

19. Integration By Parts - Exponential x Trigonometric.mp4

05:54

20. Integration by Parts - Exponential x Trigonometric - quiz.html

21. Integration By Substitution 1 Alternative to Reverse Chain Rule.mp4

09:50

22. Integration by Substitution 1 - An Alternative to Reverse Chain Rule - quiz.html

23. Integration By Substitution 2 u = f(x).mp4

10:51

24. Integration by Substitution 2 u = f(x) - quiz.html

25. Integration By Substitution 3 x = f(u).mp4

07:20

26. Integration by Substitution 3 x = f(u) - quiz.html

27. Integration By Substitution - Definite Integrals.mp4

11:09

28. Integration by Substitution - Definite Integrals - quiz.html

29. Integrating Arctan-Like Functions.mp4

06:05

30. Problem Solving With Integration.mp4

10:11

31. Problem Solving With Integration - quiz.html

32. The Trapezium Rule.mp4

15:40

33. The Trapezium Rule - quiz.html

10. Vectors

1. The Vector Equation of a Straight Line.mp4

09:01

2. Parallel and Skew Vectors.mp4

14:50

3. Converting Vector Form to Cartesian Form.mp4

04:50

4. The Scalar (Dot) Product - Introduction.mp4

08:21

5. Using the Scalar Product to Find Angles.mp4

11:19

6. Using the Scalar Product to Find Shortest Distances and Closest Points.mp4

18:06

11. Differential Equations

1. Differential Equations - Separating Variables - Part 1.mp4

14:56

2. Differential Equations - Separating Variables - Part 2.mp4

07:18

3. Differential Equations - Separating Variables - quiz.html

4. Forming Differential Equations.mp4

04:21

5. Forming Differential Equations - quiz.html

6. Modelling with Differential Equations - Part 1.mp4

10:32

7. Modelling with Differential Equations - Part 2.mp4

09:22

8. Modelling with Differential Equations - quiz.html

12. Complex Numbers

1. Complex Numbers - Introduction.mp4

11:36

2. Addition, Subtraction and Multiplication of Complex Numbers.mp4

14:45

3. Division of Complex Numbers.mp4

10:30

4. Square Roots of Complex Numbers.mp4

07:11

5. Complex Roots of Quadratic Equations.mp4

08:19

6. Complex Roots of Cubic and Quartic Equations.mp4

12:35

7. The Argand Diagram.mp4

10:05

8. Modulus and Argument.mp4

08:23

9. Modulus-Argument Polar Form of a Complex Number.mp4

11:24

10. Exponential Form of a Complex Number.mp4

09:05

11. (Optional!) Explanation of Why the Exponential Form Works.mp4

11:08

12. Multiplying and Dividing Numbers in Modulus-Argument Exponential Form - Part 1.mp4

05:29

13. Multiplying and Dividing Numbers in Modulus-Argument Exponential Form - Part 2.mp4

07:28

14. Multiplying and Dividing Numbers in Modulus-Argument Exponential Form - Part 3.mp4

09:54

15. Eulers Identity.mp4

03:04

16. Complex Loci - Circles.mp4

07:26

17. Complex Loci - Perpendicular Bisectors.mp4

07:35

18. Complex Loci - Arguments.mp4

13:04

Description

Master the content from Papers 2 & 3 (Pure Mathematics 2 & 3) of Cambridge International A-Level Maths

What You'll Learn?

Calculus
Trigonometry
Differential Equations
Complex Numbers
Vectors
Algebra

Who is this for?

People studying the Cambridge International A-Level Mathematics AS or A-Level

People who want to study an intermediate-advanced course in pure mathematics

What You Need to Know?

A good understanding of the content covered in my CIE International: A-Level Maths: Pure Mathematics 1 course, or equivalent AS maths knowledge.

More details

Description
CIE International A-Level Maths is a course for anyone studying the Cambridge International A-Level Maths:
This course covers all the pure content in Papers 2 and 3 (Pure Mathematics 2 and 3) of the Cambridge International A-Level Maths Course. It is also a great course for anyone interested in learning some more advanced pure maths.

The main sections of the course are:
- Algebra - we'll learn about the modulus function and how to solve equations and inequalities using it, as well as polynomial division, remainders and the factor theorem.
- Logarithms and Exponentials Functions - we'll learn what these are, and how to use them to solve many different styles of problems. We'll also learn about e^x and the natural logarithm.
- Trigonometry - we'll learn three new trig functions (sec, cosec and cot) as well as explore many new trigonometric identities, including double angles.
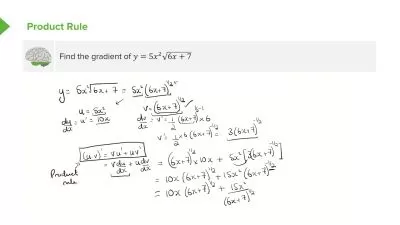
- Differentiation - we'll learn how to different almost any function you can think of! We'll use the chain, product and quotient rules and also look at implicit and parametric differentiation.
- Integration - we'll learn a range of techniques to integrate most functions we've seen so far.
- Numerical Solutions to Equations - We'll explore ways of finding approximate solutions to equations with no analytical solutions, including iteration.
- Further Algebra - we'll learn how to split algebraic fractions into partial fractions, and how to extend binomial expansion to include negative and fractional powers.
- Further Integration - We'll extend what we've already covered, and look at reverse chain rule, substitution and integration by parts.
- Vectors - we'll learn how to work with vectors in 3d and how to use the vector equation of straight lines and the scalar (dot) product.
- Differential Equations - we'll explore how to set up and solve differential equations, and how to apply these in real world contexts.
- Complex Numbers - we'll explore the fascinating world of complex numbers, including the argand diagram, polar and exponential forms, and complex loci.

Those of you studying for Paper 3 will cover all of these section. If you're studying for Paper 2, you will study up to Numerical Solutions to Equations.

Please note: This course is intended for people studying the Cambridge International A-Level Maths Syllabus, and not the UK syllabus (covered by Edexcel, OCR, AQA and MEI exam boards). If you are looking for these, check out my other courses on these!

What you get in this course:
Videos: Watch as I explain each topic, introducing all the key ideas, and then go through a range of different examples, covering all the important ideas in each. In these videos I also point out the most common misconceptions and errors so that you can avoid them.
Quizzes: Each sub-section is followed by a short quiz for you to test your understanding of the content just covered. Most of the questions in the quizzes are taken from real A-Level past papers. Feel free to ask for help if you get stuck on these!
Worksheets: At the end of each chapter I have made a collection of different questions taken from real A-Level past papers for you to put it all together and try for yourself. At the bottom of each worksheet is a full mark-scheme so you can see how you have done.

This course comes with:
Â· A printable Udemy certificate of completion.
Â· Support in the Q&A section - ask me if you get stuck!

I really hope you enjoy this course!
Woody
Who this course is for:
People studying the Cambridge International A-Level Mathematics AS or A-Level
People who want to study an intermediate-advanced course in pure mathematics

User Reviews

Rating

average 0

Total votes0

Focused display

Math

Woody Lewenstein

Instructor's Courses

After finishing my studies at Oxford University I worked for a year in India before moving to London, which is where I have been ever since.I have taught mathematics in some of the best performing schools in the country for over 10 years, where I have taught all levels of school maths, including GCSE, A-Level, Further Maths and Oxbridge entrance paper preparation. I also have 1000's of hours of tutoring experience working one-to-one with students on A-level maths and further maths.Alongside this I have worked with businesses to train their staff in mathematical skills, such as statistics, data analysis and mathematical software packages.Away from my work I love music and long-distance cycling, and recently cycled from London to Istanbul.

Udemy

View courses Udemy

Students take courses primarily to improve job-related skills.Some courses generate credit toward technical certification. Udemy has made a special effort to attract corporate trainers seeking to create coursework for employees of their company.